Ever felt like banging your head against a wall as you’re teaching this one? You’re in good company!

Usually, we go to that old procedure we were taught where you underline the place you’re rounding to (if you’re rounding to the nearest 10, you underline the 10s place), then look at the place next to it – if it’s 5 or higher you round up, if it’s 4 or lower, you round down. Oh, and don’t forget to add zeros and leave all the other numbers the same! Crystal clear, right???
This definitely falls into the category of kids memorizing a procedure for a concept they don’t understand. I was in a third grade classroom where the teacher had students in a Math with the Teacher station, and they were learning about rounding up or down. The students seemed to be doing well while they were in the station because she was there guiding them, but when they went back to their seats, they were really struggling.
Some kids could use a number line and plot the two points, find the midpoint, and then determine whether the number is closer to 150 or 160. But not all students have the number sense to be able to make that decision.
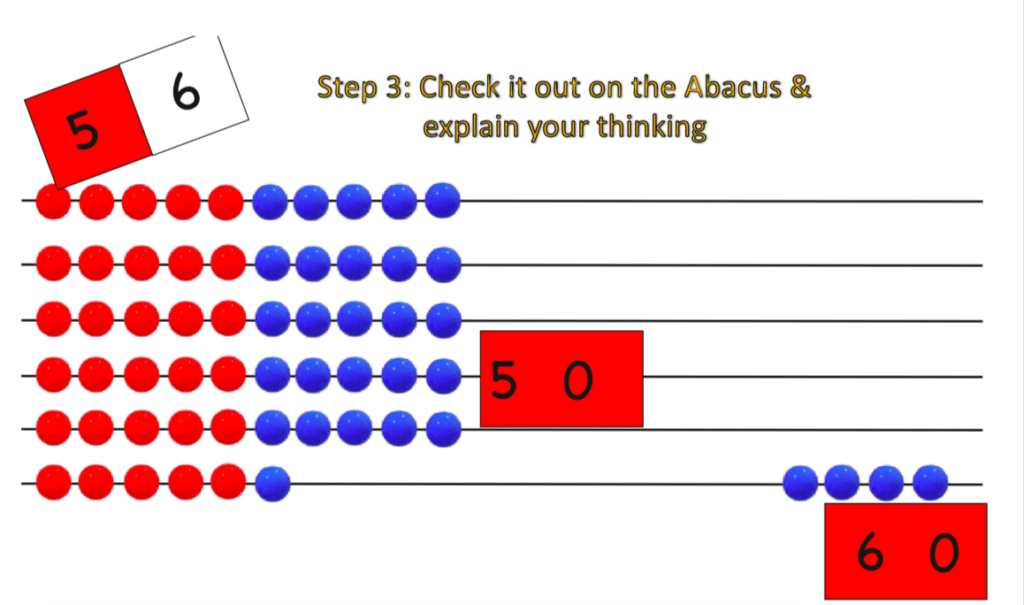
An abacus is another great option for this situation, and so I grabbed an abacus (which I unwrapped because it was still shrink-wrapped at the back of the classroom!), and sat down with one student to take a closer look at his number – 156. We were supposed to round to the nearest 10, so would it go up to 160 or down to 150?
Let’s think of the number 156. You could build it with two abaci – one to show the full 100 and the other to show 56 – but we just used the one abacus to show 56 as a number between 50 and 60. Once it was built, I asked, “Is it quicker for you to push all 6 beads back and go to 50? Or should we just push 4 beads forward and go up to 60?” Obviously, he told me that it would be much quicker to push 4 beads and go up to 60, so he knew that he would round 156 up to 160.
I did a couple problems with him in this way, and finally let him take the abacus to do some problems on his own. Using the visual representation on the abacus, he totally got the idea of rounding and was able to explain to me why he would round up or down.
The best part? Afterwards, the student said, “Is that what they were trying to explain with the five and higher four and lower round up keep the same stuff?? This way is way easier, Miss Shannon!” Some kids can’t do it abstractly, or pictorially (which is what rounding on a number line would be), so they need the concrete visual of the abacus to be able to see exactly what they’re thinking in their head.
What about students who can already do rounding? They need to be able to do this too! It’s important for them to be able to articulate the why behind what they’re doing and why they made a decision to round the way they did.
This set of PowerPoint presentations and video tutorials on rounding ranges from rounding to the nearest 10 within 100, to the nearest 100 (2nd grade), to the nearest 10 within 1000 (4th grade), and rounding with decimals (5th).
In these videos, we’ll offer both the abacus and the number lines as options for students to use as they work to understand the concept and visually see how they’re rounding.
Using a number line, as you’ll see in the videos, is all about finding the midpoint. Students will plot their two points, say 150 and 160 on the number line, find the midpoint (you can use the abacus to help them find this!), and then plot the number in question. Then, it’s a simple decision of which endpoint is closer.
Rounding with Decimals
If rounding with whole numbers is confusing, rounding with decimals can quickly become really confusing for students. If you ask them to round 5.26 to the nearest 10th, kids have to think the fact that we’re trying to round between two tenths and three tenths. The big question is, do your students know where the midpoint is in a problem like this? If they put 5.2 and 5.3 on a number line, could they identify the midpoint as 5.25? Many times, students don’t have the number sense for this and so, we can use the place value discs as a really great way for kids to visualize how those numbers go.
Once they find that midpoint, they then have to decide: Is 5.26 closer to 5.2 or 5.3? Again, kids being able to find that midpoint and be able to plot that number will really help them to be able to make that decision. There’s certainly nothing wrong with using the abacus as an alternative to represent what they’re trying to figure out. Make the single row of the abacus beads represent 10ths, so each of those beads would be worth one 10th.You could also use the entire abacus and have each bead be worth one 100th.
Make it Concrete
Place value is a very abstract concept for kids, and these tutorial videos will help make both more/less and rounding concepts more concrete. It’s so important for students to have a firm grasp on both concepts. If a student can get the idea of rounding, it will help them when they come to estimating and some of the other higher level math concepts. So often, math books only give you a few days for rounding, but in reality, kids need so much more repetition.
Try a rounding exercise as a warm up, even if you’re teaching virtually! Use a Google Doc or Slide to have students plot points on a number line. Or use a Flip Grid video to have students explain why they chose to round one way or another.
Don’t forget that having students explain their thinking is just as important as getting the right answer, if not more so!
Check out our Place Value: Rounding series (M³ Members – you can download it for free!) and let us know what you think! Use the videos to help kids (and even parents!) understand how to explain the rounding process!

K-5 Math Teaching Resources has some great games in the 2nd and 3rd levels to help kids get some extra practice with rounding, and M³ Members, check out the tutorial videos and accountability sheets that go with them!